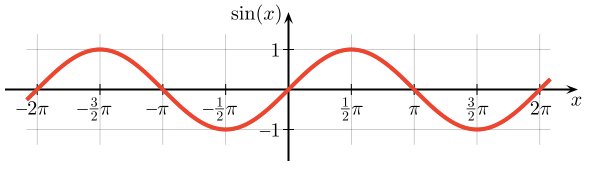
First, a quick intro to sine wave.
Y = sin(x) or
Y = A sin(2pi*n*t)
A- amplitude
n- frequency
t - time
The above function describes (a graph) a simple and neat sine curve as the time progress.
Take two such waves and add them or superimpose one on the other(interference).
Mathematically
Y = A sin(2pi*n*t)+ A sin(2pi*n*t)
Y = (A+A) sin( 2pi*n*t)
Here the amplitude doubles. We get a bigger wave.
Now subtract both the waves.
Y = A sin(2pi*n*t) - A sin(2pi*n*t)
= 0
The waves cancel each other. Figures are given above.
Take two sine waves of slightly different frequencies and overlap them.
Y = A sin(2pi*n*t)+ A sin(2pi*(n+m)*t)
m = 1 or 2
If one draws a graph for the above function, a beautiful wave pattern results. It is called beats. Suppose, the two waves are sound waves and they overlap, beats arises. Beats is periodic waxing and waning of the sound. The wave pattern clearly indicates this.
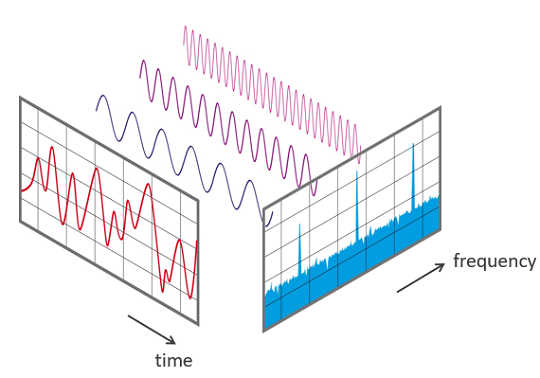
Now we understand that by adding sine waves, one can produce any complex wave pattern. By extending the argument, we can say, any periodic wave can be split up into sine waves of different frequencies. For example, a square wave can be broken down as follows.
Any wave can be split up into its sine wave components as illustrated below.
"Splitting a complicated waves into its sine wave or cosine wave components of different frequencies" is called Fourier transformation. The experts say that it is bringing the wave from time domain to frequency domain.
There are formulas, procedures, software, tools, website to do this transformation effectively.
What is the use: The voice, picture and video all can be represented in wave forms. They can be Fourier-transformed electronically. Once we get the waves in frequency domain,
1. We can compress, store and transmit them with less resources.
2. Unwanted noise -certain frequencies can be eliminated easily.
Any wave form can be analysed with this mathematical tool. It plays an important role in science, engineering and medicine. It is an important invention in the history of mathmatics.
Note: Even your signature can be taken as waveform. It also can be analysed with Fourier's tool.
Y = sin(x) or
Y = A sin(2pi*n*t)
A- amplitude
n- frequency
t - time
The above function describes (a graph) a simple and neat sine curve as the time progress.
Take two such waves and add them or superimpose one on the other(interference).
Mathematically
Y = A sin(2pi*n*t)+ A sin(2pi*n*t)
Y = (A+A) sin( 2pi*n*t)
Here the amplitude doubles. We get a bigger wave.
Now subtract both the waves.
Y = A sin(2pi*n*t) - A sin(2pi*n*t)
= 0
The waves cancel each other. Figures are given above.
Take two sine waves of slightly different frequencies and overlap them.
Y = A sin(2pi*n*t)+ A sin(2pi*(n+m)*t)
m = 1 or 2
If one draws a graph for the above function, a beautiful wave pattern results. It is called beats. Suppose, the two waves are sound waves and they overlap, beats arises. Beats is periodic waxing and waning of the sound. The wave pattern clearly indicates this.
Now we understand that by adding sine waves, one can produce any complex wave pattern. By extending the argument, we can say, any periodic wave can be split up into sine waves of different frequencies. For example, a square wave can be broken down as follows.
Any wave can be split up into its sine wave components as illustrated below.
"Splitting a complicated waves into its sine wave or cosine wave components of different frequencies" is called Fourier transformation. The experts say that it is bringing the wave from time domain to frequency domain.
There are formulas, procedures, software, tools, website to do this transformation effectively.
What is the use: The voice, picture and video all can be represented in wave forms. They can be Fourier-transformed electronically. Once we get the waves in frequency domain,
1. We can compress, store and transmit them with less resources.
2. Unwanted noise -certain frequencies can be eliminated easily.
Any wave form can be analysed with this mathematical tool. It plays an important role in science, engineering and medicine. It is an important invention in the history of mathmatics.
Note: Even your signature can be taken as waveform. It also can be analysed with Fourier's tool.